Multi_R_Designer Tutorial

---

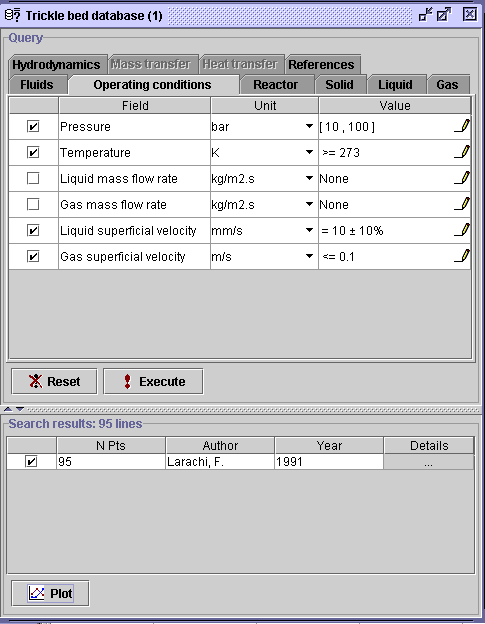
1) Open the file (Case problem I.mr) as it was saved in Case problem I. In the Hydrodynamics tab, unselect Total pressure drop and select Frictional pressure drop. Press the Execute button. The following Query panel containing the reduced Search results list in accordance with the problem specifications is opened.
 

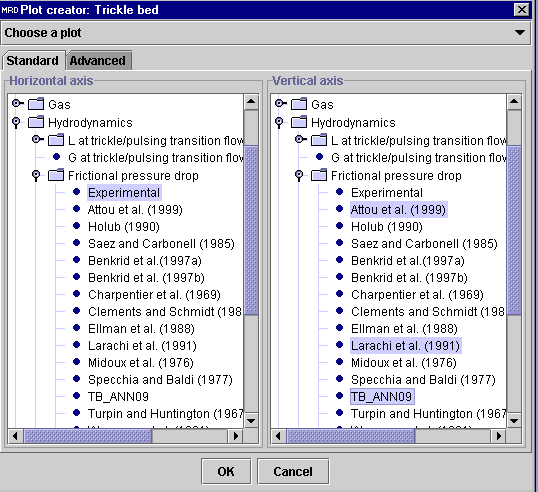
2) Select the Plot button  to enable the plot creator.

3) In the Horizontal axis panel (Standard tab), click on the knob corresponding to Hydrodynamics, then on the knob corresponding to Frictional pressure drop. Highlight Experimental.

4) In the Vertical axis panel, click on Hydrodynamics, then on Frictional pressure drop. Highlight Attou et al.(1999), Larachi et al. (1991) and TB_ANN09.

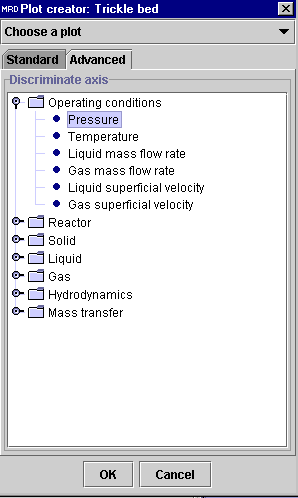
5) In the Advanced tab, click on Operating conditions and highlight Pressure. Press OK. The following diagram will be created.
 

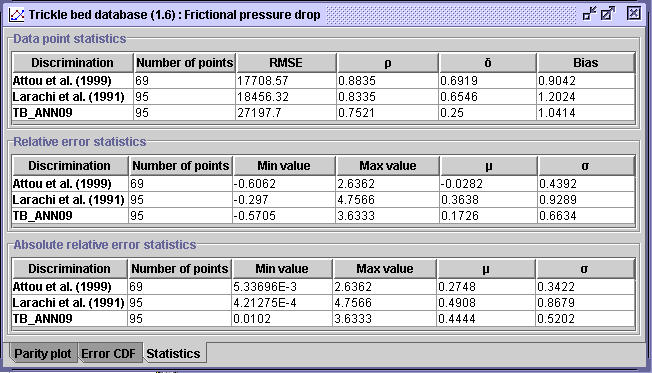
6) The default envelopes (RMSE envelopes) is  based on the first model selected in the Plot creator i.e. Attou et al. model.
 

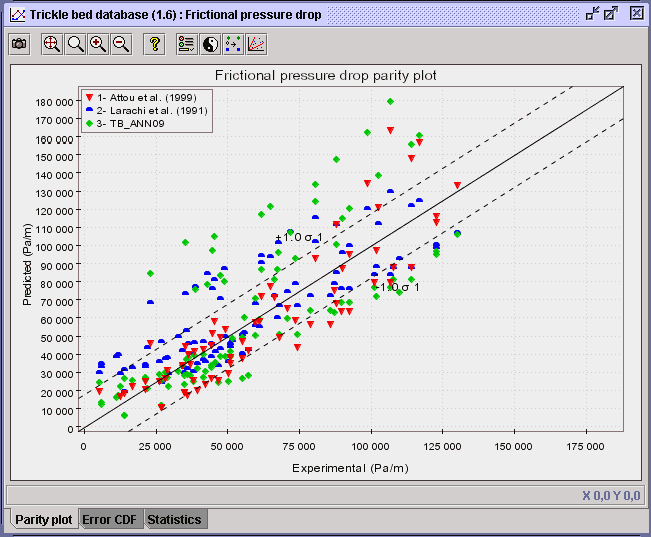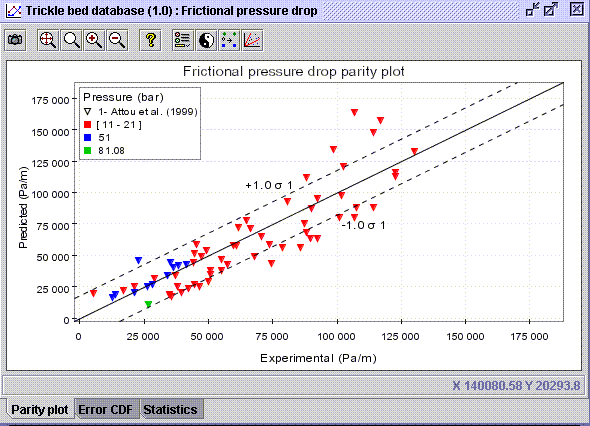
7) The three models seem to give an equally acceptable prediction of the frictional pressure drop. A  more accurate analysis could be obtained from the Statistics table.The Attou et al. model is applicable only on 69 points (low interaction flow regime) while the other two correlations are applied on the 95  experimental data sets.
 
 

CONCLUSION #1:  The Attou et al. (1999) model clearly predicts the best the frictional pressure drop with the lowest AARE (0.275) and the highest correlation coefficient (=0.88). This conclusion changes from Conclusion #1 in Sub-problem 6.1, where the TB_ANN09 correlation presented much better qualities based on the whole databank for frictional pressure drop. For further analysis, it would interesting to discriminate the current plot in function of pressure since its range is wide enough [10-100 bars] to create several sub-divisions.

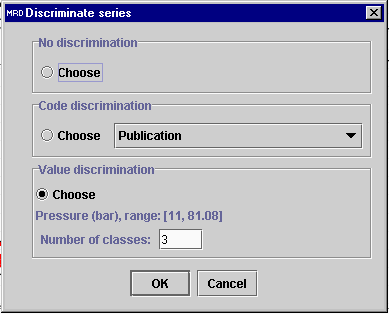
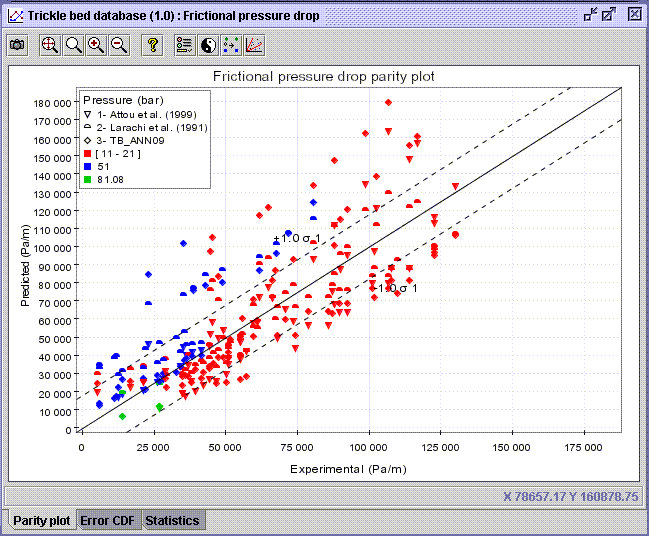
8) Select the Discriminate series  icon. Activate the Value discrimination option, and write 3 in the Number of classes entry. Press OK. The following diagram with a double discrimination based on the correlations and pressure sub-divisions is presented to the user (N.B. After a discrimination, the envelopes do not change).
 

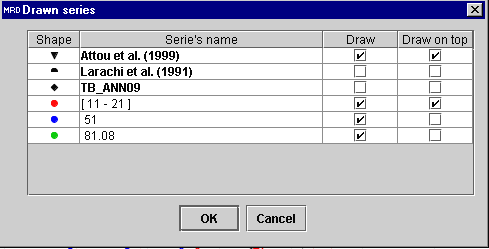
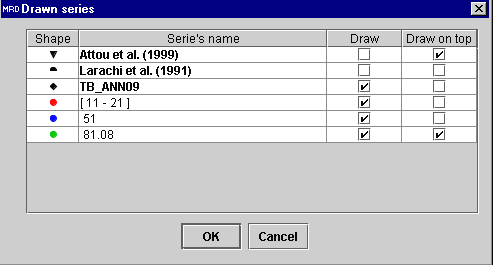
9) Right click on the legend. The following dialog window offers the possibility to remove some series. Unselect in the Draw field, the Larachi et al. (1991) and TB_ANN09 correlations while keeping all the pressure sub-divisions. Press OK.
sa
Comment: The Draw on top field assigns a serie to be drawn over the others for better view if necessary. In this case, the [10-21] sub-division applied on the Attou et al. (1999) model is drawn on top.
 

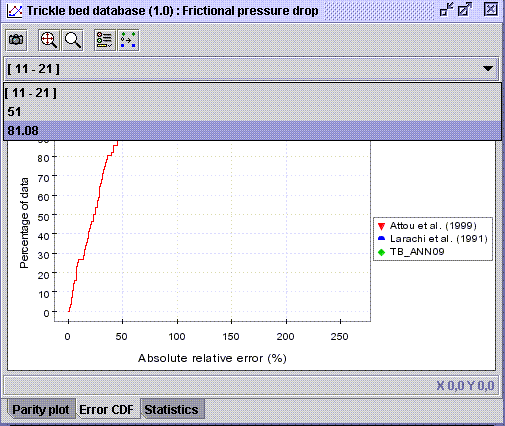
10) When discriminating, a combo box  in the Error CDF panel which contains the discriminating series title appears. In this case, 3 choices are offered to the user and the CDF curve can drawn for either one of them.
aa
Comment: As mentioned previously, the Parity plot and Error CDF panels are inter-connected in many ways. As a consequence, the TB_ANN09 and Larachi et al. (1991) correlations, which were withdrawn from the parity plot, are not presented on the CDF curves. Generally, the CDF curves are generated when the corresponding serie in the parity plot is shown.
 

11) When discriminating, new fields are added in the Statistics panel. For each correlation, the statistics based on every predictions without considering the discrimination and invalidity specifications are offered. Then, the statistics exclusively associated with the Shown points in the parity plot are also given. For Attou et al. (1999), 69 points are actually shown on the parity plot while the other two correlations, which were temporally withdrawn from the plot, presents empty cells. Finally, the discriminated series (sub-divisions of the Shown points) statistics gives a good look on the capacity of the model to estimate frictional pressure drop for specific operating conditions.
 

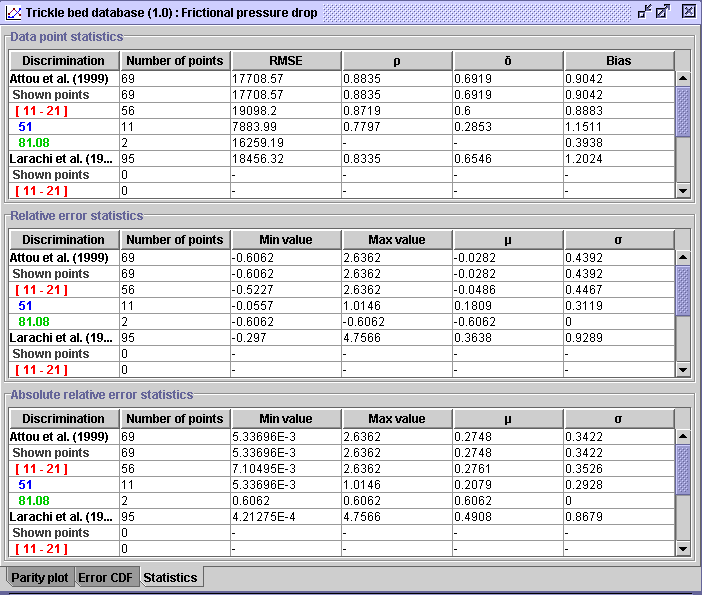CONCLUSION #2: The Attou et al. (1999) model seems more accurate for pressures surrounding 51 bars. For the 11 studied data in this range, the correlation manifests an Average Absolute Relative Error (AARE) of ca. 20% while the predictions for pressures near 81 bars are slightly off-track (AARE=60%).

12) To analyze the TB_ANN09 correlation, right click on the legend box. Unselect Attou et al. (1999) and re-select TB_ANN09. Press OK.
 

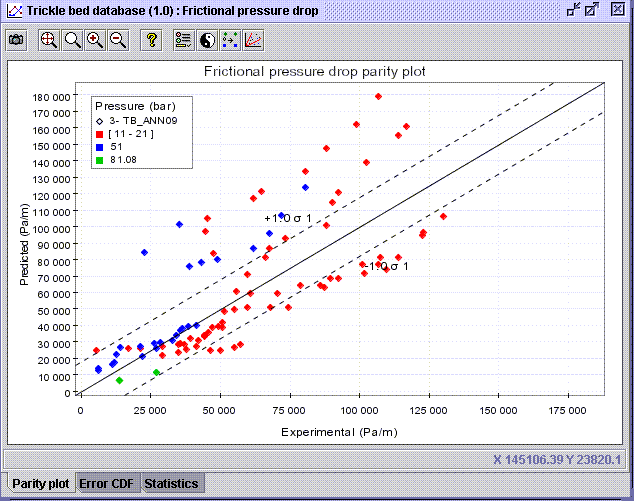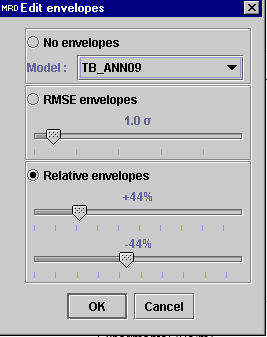
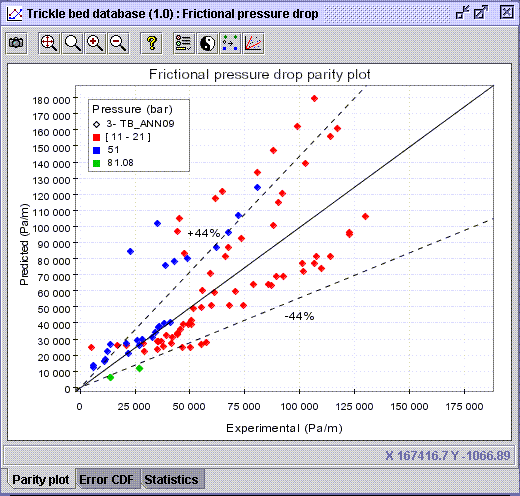
13) The envelopes have disappeared since it was set up for the Attou et al. (1999) correlation. Return in the Edit envelopes  panel. In the combo box , choose TB_ANN09. It has automatically switched to the Relative envelopes option and adjusted the envelopes to +35% and -35%, which corresponds to the average absolute relative error (AARE) of the TB_ANN09 correlation. Press OK.
 

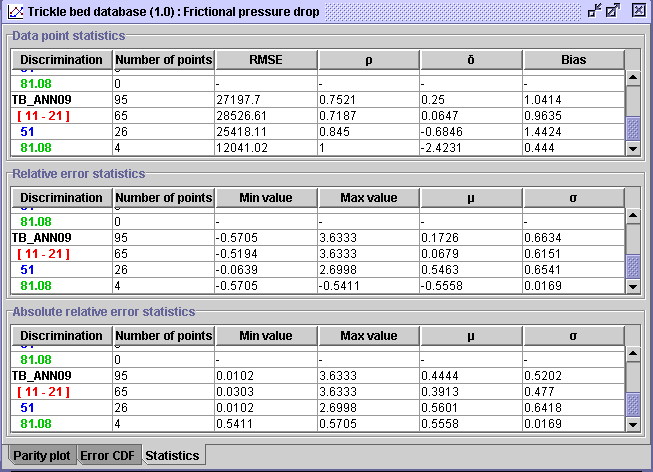
14) In the Statistics panel, only the TB_ANN09 correlation contains statistical values. First, it attest that all studied data are valid (TB_ANN09 Points = Shown points = 95).  The data repartition is given as follows: Pressure [11-21] bars - 65 experiments; Pressure [51] bars - 26 experiments; Pressure [81.1 bars - 4 experiments.
 

15) To compare, simultaneously, the performance of the three model/correlation, right click in the legend box and select Attou et al. (1999) and Larachi et al. (1991).
The following statistics are then obtained:
 
 

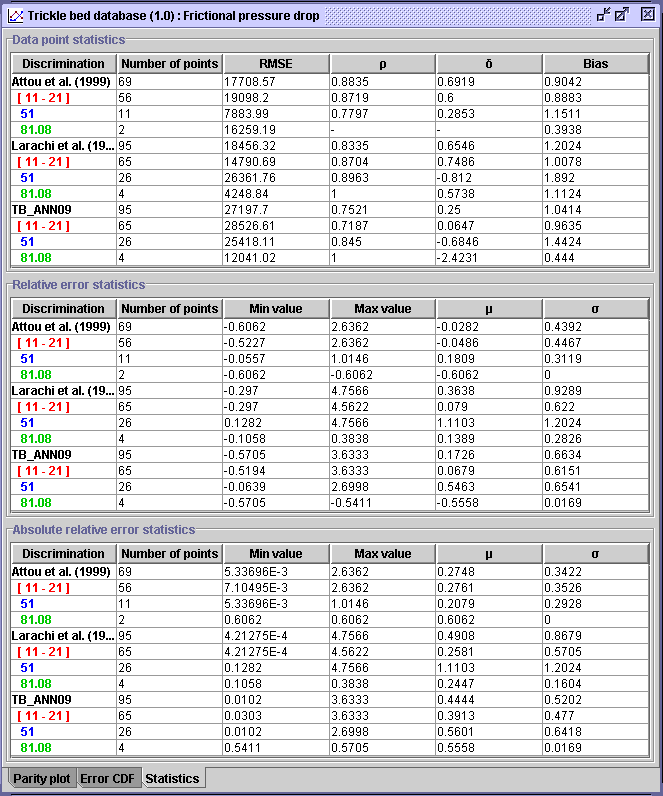CONCLUSION #3: Generally speaking, the TB_ANN09 correlation presents good predicting capability for pressure 51 bars with an AARE of 56%. The Larachi et al. (1991) correlation predicting frictional pressure drop is best for operating pressures of 10-20 bars, while for cases reaching pressures higher than 50 bars either of them can be used depending on the criteria used : Absolute Relative Error () or standard deviation ()

Multi_R_Designer Tutorial